ENGINEERING MECHANICS 373

EXTRA CREDIT PROJECT

Compiled by: Allen Roelfs

For: Dr. M. Negahban

December 4, 2002

For my project, I chose to model the collision of three objects in a horizontal plane. This example is quite similar to imagining three hockey pucks, all of equal mass, on a sheet of ice with little or no friction acting between themselves and the surface of the ice. As the first puck is hit, it is given an initial velocity of V(o-a) and it inevitably strikes puck two. How do we relate the final velocity of puck “a” and the initial velocity of puck “b”?

The answer lies in a characteristic of each collision known as the coefficient of restitution. Simply put, the coefficient of restitution is the amount of “bounciness” there is between two objects in a collision and tells us how the final velocity of each object will be determined. The relation between velocities and the coefficient of restitution is given as

V’a -V’b = e (Va – Vb), were “e” is the coefficient of restitution and the symbol prime indicates final velocity.

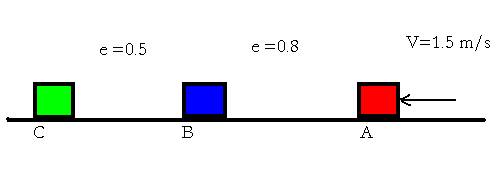
In this example, all blocks have equal mass, and block “A” has initial velocity 1.5 m/s to the left. The coefficient of restitution for each collision is shown. The derivation of each initial and final velocity goes as follows:

MaVa + MbVb = MaV’a + MbV’b

Va = V’a + V’b

1.5 = V’a + V’b

V’a = 1.5 – V’b

V’a – V’b = e(Vb - Va)

1.5 – V’b – V’b = 0.8(-1.5)

-2(V’b) + 1.5 = -1.2

2.7 = -2(V’b)

V’b (initial) = 1.35 m/s

V’c = 1.5 – 1.35

V’a = 0.15 m/s

Vb + Vc = V’b + V’c

Vb = V’b + V’c

1.35 = V’b +V’c

V’b = 1.35 – V’c

V’b – V’c = e (Vc – Vb)

1.35 – V’c – V’c = 0.5 (-1.35)

1.35 – 2(V’c) = -.675

2.025 = 2 (V’c)

V’c = 1.0125 m/s

V’b = 1.35 – 1.0125

V’b (final) = 0.3375 m/s

The results of this derivation are confirmed by examining the working model

and observing the velocity indicators on the page.

To give a better idea of a slightly more practical application of the

coefficient of restitution, I have prepared another working model of a collision between a truck and a tree occurring at excessive highway speeds. In this simulation, you will notice how the truck responds with very little elasticity to the tree. This is due to the low value of the coefficient of restitution due to the composition of the truck, being mostly steel. To view this simulation, click here.

I hope you have found this short explanation of momentum conservation and the effects of the coefficient of restitution on colliding particles useful in your study of dynamics.

Allen Roelfs