1-D Integration and Centroids
Integral of a function:
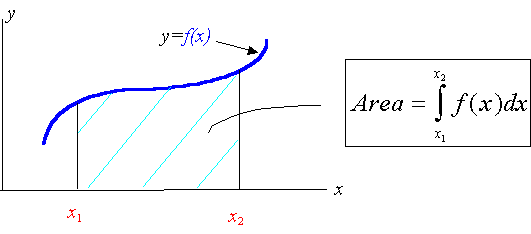
The integral of a function f(x) over an interval from x1 to x2 yield the area under the curve in this intervalNote: The integral represents the as
.
Indefinite Integrals to know []:
Note:
Remember to add a constant of integration if you are not specifying limits. You evaluate the constant of integration by forcing the integral to pass through a known point.Note:
For definite integrals subtract the value of the integral at the lower limit from its value at the upper limit. For example, if you have the indefinite integralwhere C is the constant of integration which drops out of the final expression.
Note:
The following notation is commonIntegration by parts:
Centroid of an area:
The centroid of an area is the area weighted average location of the given area.Centroids of common shapes: