The angular impulse-momentum relation

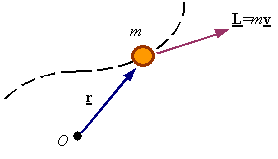
Angular momentum of a particle: The symbol H_O denotes the angular momentum and is defined as the moment of linear momentum around the point O, and given by the equation

Example 1

Example 2

Impulse of a moment from time t₁ to t₂: The integral of the moment over the time interval of concern is its impulse. The impulse of a moment is a vector given by the integral

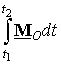
Moment and rate-of-change-of angular momentum relation: Starting from Newton’s second law, calculating the moment of both sided of the equation with respect to a point O on an inertial frame results in

This resulting relation between the moment and the rate of change of angular momentum is called the moment-rate of change of angular momentum relation (MRCAM).

Example 3

The angular impulse-momentum relation: Integration of Newton’s 2^nd law over the time interval from t₁ to t₂ results in

Therefore, the angular momentum of a particle is changed by the impulse of the resultant moment on the particle. There will be conservation of angular momentum only if the impulse of the resultant moment is zero.

Example 4

ã Mehrdad Negahban and the University of Nebraska, 1996-2002.

All rights reserved

Copy and distribute freely for personal use only

Department of Engineering Mechanics, University of Nebraska, Lincoln, NE 68588-0526