CALCULUS

Areas of Focus:

1. Differentiation
2. Maximization and minimization
3. Partial derivatives
4. Integration
5. Integration over a line
6. Double integrals
7. Integration over an area
8. Centroid of an area
9. Integrating differential equations

Differentiation:

The derivative of a function is a measure of how the function changes as a result of a change in the value of its argument. Given the function f(x), the derivative of f with respect to x is written as or as 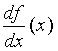, and is defined by

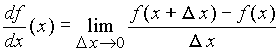
As shown in the figure, the derivative of the function f(x) at point x gives the slope of the function at x in terms of the ratio of the rise divided by the run for the line AB that is tangent to the curve at point x.

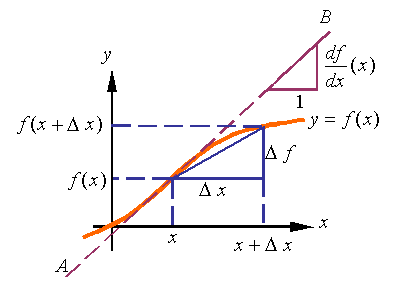
The derivative of the function f(x) is also sometimes written as f ' (x). As shown in the figure, one can also write the definition of the derivative as

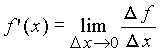
The basic rules of differentiation are

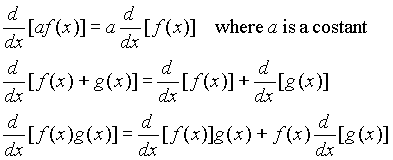
The derivative of commonly used functions:

The following is a list of the derivatives of some of the more commonly used functions.

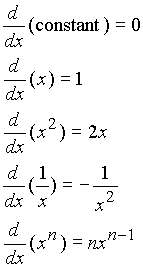
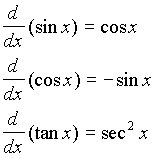
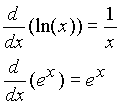
The product rule for derivatives:

Consider a function such as f(x)=g(x)h(x) that is the product of two functions. The product rule can be used to calculate the derivative of f with respect to x. The product rule states that

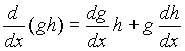
For example, To take the derivative of f(x)=(2x+3)(4x+5)² one can follow these steps

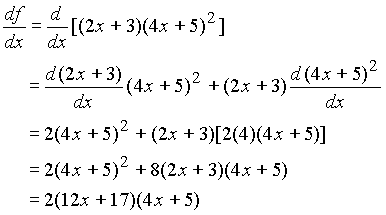
The chain rule for derivatives:

Consider the function f(U), where U is a function of x. One can calculate the derivative of f with respect to x by using the chain rule given by

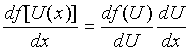
For example, to calculate the derivative of f(U) = Uⁿ with respect to x, where U = x²+a, one can follow these steps

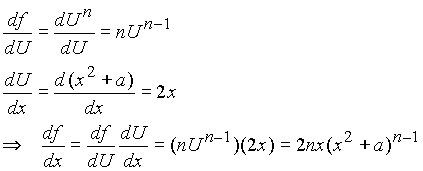
Maximization and minimization:

A point on a smooth function where the derivative is zero is a local maximum, a local minimum, or an inflection point of the function. This can be clearly seen in the figure, where the function has three points at which the tangent to the curve is horizontal (the slope is zero). This function has a local maximum at A, a local minimum at B, and an inflection point at C.

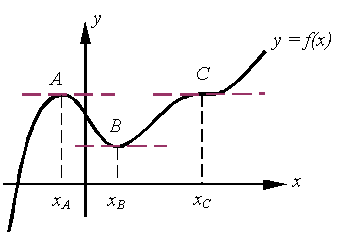
One can determine whether a point with zero derivative is a local maximum, a local minimum, or an inflection point by evaluating the value of the second derivative at that point.

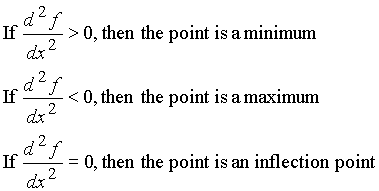
Given a smooth function f(x), one can find the local maximums, local minimums, and inflections points by solving the equation

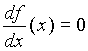
to get all points x that have a derivative of zero. One can then check the second derivative for each point to get the specific character of the function at each point.

Global maximum and minimum:

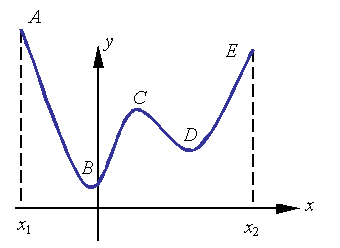
The global maximum or minimum of a smooth function in a specific interval of its argument occurs either at the limits of the interval or at a point inside the interval where the function has a derivative of zero. As can be seen in the figure, the function shown has a global maximum at point A on the left boundary of the interval under consideration, a global minimum at B, a local maximum at C, and a local minimum at D.

Partial derivative:

The derivative of a function of several variable with respect to only one of its variables is called a partial derivative. Given a function f(x,y), its partial derivative with respect to its first argument is denoted by and defined by

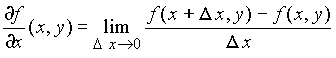
Since all other variables are kept constant during the partial derivative, it represents the slope of the curve one obtains when varying only the designated argument of the function.

For example, the partial derivative of the function with respect to x is evaluated by treating y as a constant so that one gets

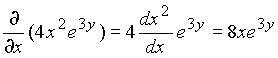
The chain rule:

Consider a function f[U(x), V(x)] of two arguments U and V, each a function of x. The chain rule can be used to find the derivative of f with respect to x by the rule

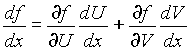
For example, consider the function f = UV² where U =2x+3 and V=4x². The derivative of f with respect to x is given by

Integration:

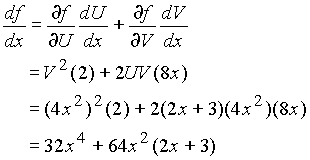
The integral of a function f(x) over an interval from x₁ to x₂ yields the area under the curve of the function over this same interval.

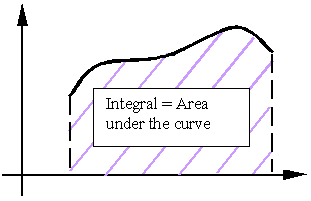
Let F denote the integral of f(x) over the interval from x₁ to x₂. This is written as

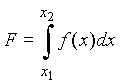
and is called a definite integral since the limits of integration are prescribed. The area under the curve in the following figure can be approximated by adding together the vertical strips of area . Therefore, the integral is approximated by

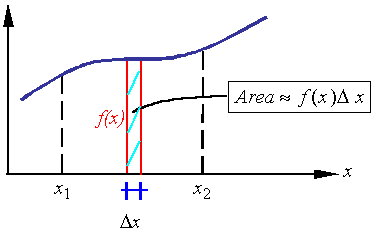
This approximation approaches the value of the integral as the width of the strips approaches zero.

Indefinite Integrals:

A function F(x) is the indefinite integral of the function f(x) if

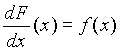
The indefinite integral is also know as the anti-derivative. Since the derivative of a constant is zero, the indefinite integral of a function can only be evaluated up to the addition of a constant. Therefore, given a function F(x) to be an anti-derivative of f(x), the function F(x) + C, where C is any constant, is also an anti-derivative of f(x). This constant is known as the constant of integration and may be determined only if one has additional information about the integral. Normally, a known value of the integral at a specified point is used to calculate the constant of integration.

The basic rules of integration are

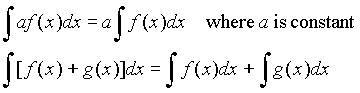
The indefinite integral of commonly used functions:

The following is a list of indefinite integrals of commonly used functions, up to a constant of integration []:

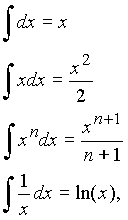
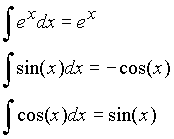
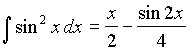
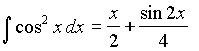
Note: Remember to add a constant of integration. You evaluate the constant of integration by selecting the constant of integration such that the integral passes through a known point.

Relating definite and indefinite integrals:

To obtain the value of the definite integral knowing the value of the indefinite integral of the function, one can subtract the value of the definite integral evaluated at the lower limit of integration from its value at the upper limit of integration. For example, if you have the indefinite integral

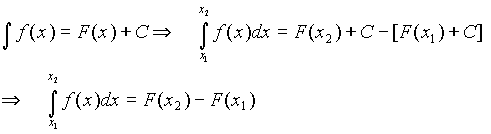
Note that C, the constant of integration, cancels in the subtraction and need not be included. It is common to sometimes use the notation

Change of variables:

Given a function U(x), one can use 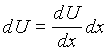to change the variable of integration from x to U . The change of variables results in the rule

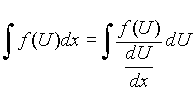
For example, given the function 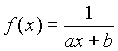we can write this function as where U = ax+b and 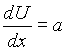. Therefore, the integral of f can be evaluated by using the following steps.

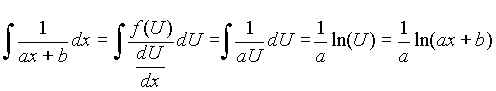
Change of variables for a definite integral is similar with an additional change in the limits of integration. The resulting equation is

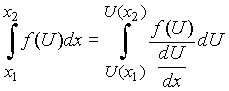
For example, given the function , we can write this function as where U = ax and 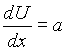. Therefore, the integral of f can be evaluated by using the following steps.

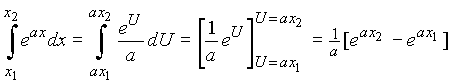
Integration by parts:

Given the functions U(x) and V(x), one can use integration by parts to integrate the following integral using the relation

For example, to evaluate the integral

one can take and so that and . Using integration by parts we get

Integration over a line:

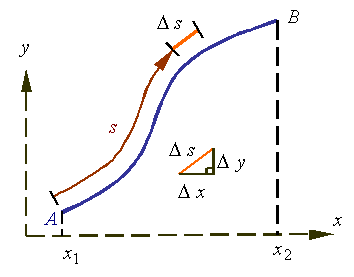
The integral F of function f(s) over line AB, that is defined by s = 0 to s = l, is written as

.

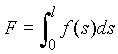When either the domain of integration or the function is described in terms of another variable, such as x in the figure, one can evaluate F by a change of variables to get

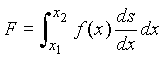
Depending on the format the information is provided in, it might be necessary to use the Pythagorean Theorem to relate the differential line element along the arc of the curve to the x and y coordinates. For example, in the figure shown we can see that

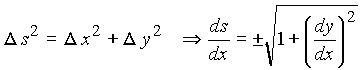
The sign of the root must be selected based on the specifics of the problem under consideration.

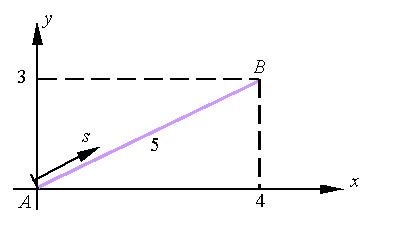
For example, consider integrating the function f(x,y) =xy² over the straight line defined in the figure from point A to point B. Direct integration, using the relations

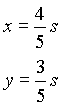
would yield

Double integral:

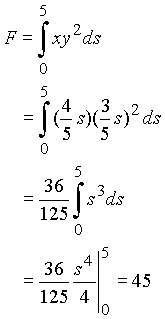
The double integral of function f(x,y) first integrating over x and then integrating over y is given by

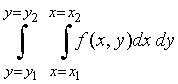
The notation implies that the inner integral over x is done first, treating y as a constant.

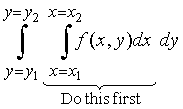
Once the inner integral is completed and the limits of integration for x are substituted into the expression, the outer integral is evaluated and the limits for y are substituted into the resulting expression. The rules of integration are the same as used for single integration for both the integration over x and the integration over y. To integrate over y first and then over x, the integration would be written as

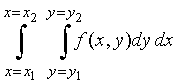
One can also write the integral limits without specifying the variable (i.e., without using "x=" and "y="). The order dxdy or dydx clearly specifies what variable a specific limit is associated with.

Consider the following example of double integration of the function f(x,y) =xy².

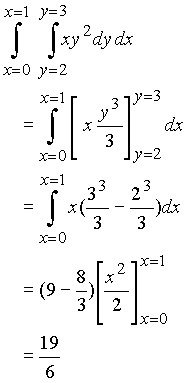
Unlike the example, the limits of integration need not be constants. There will be no problem as long as the inner integral is conducted fist and the limits are substituted into the resulting expression before the outer integral is evaluated. For example, consider the following integral.

Integration over an area:

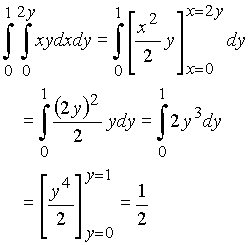
The double integral F of the function over the area A is written as

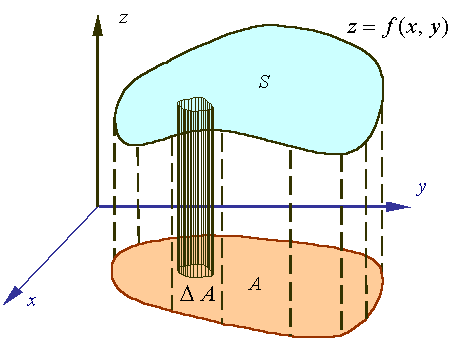
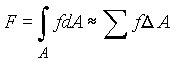
This integral is the sum of fdA over the area A. Each fdA is the volume of the column with base dA and height f. Thus, the integral gives the volume under the surface . To accomplish the summation that is represented by the integral, one needs to section the domain of integration A into small parts (i.e., into many small dAs). As shown in the figure, the domain of integration can be sections into rectangular sections, each with an area . At the limit of small element sizes, the sum of these area elements adds up to the original domain of integration.

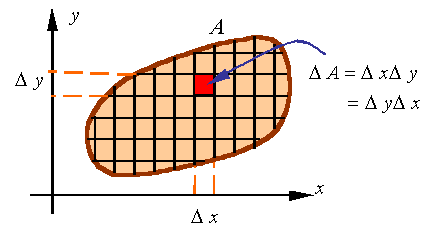
 The differential element of area dA in a rectangular x-y coordinate system is given by either dA=dxdy or dA=dydx. The difference between the two is the order of integration. If done correctly, the value of the integration does not depend on this selection, yet the ease of integration may strongly depend on the choice for dA. If dA=dxdy is selected, then for each value of y an integration over x is conducted from the left limit of the domain to its right limit. This process fills the domain with differential elements of area and is shown in the figure.

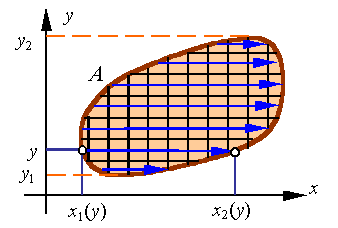
As can be seen from the figure, the limits of integration of x depend on the value of y so that the integral is written as

On the other hand, if one takes dA=dydx, the order of integration changes. In this case, for each x between x₁ and x₂, the argument is integrated over y from the lower limit of area to its upper limit. This is shown in the figure.

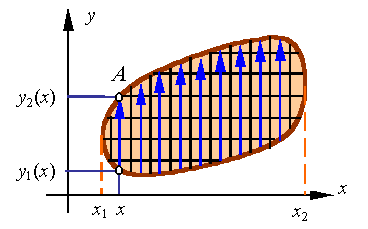
In this case the integral can be written as

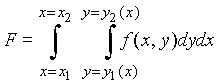
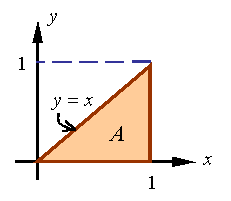
For example, consider the integral of f(x,y) =xy over the domain shown in the figure.

The integral can be defined as

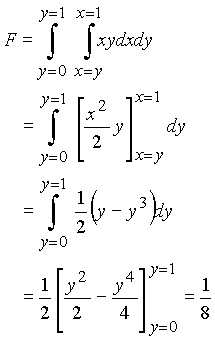
Alternately, one can get the integral from

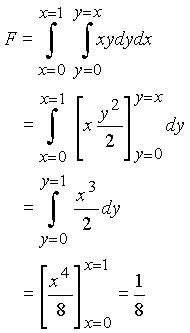
As can be seen, the result of the integration does not change based on the selection of the order of integration, yet the setup of the integrals does change.

Polar coordinates:

The differential element of area in polar coordinates is given by

This is a result of fact that the circumferential sides of the differential element of area have a length of , as shown in the figure. Otherwise the integration process is similar to rectangular coordinates.

Centroid of an area:

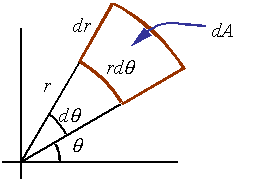
The centroid of an area is the area weighted average location of the given area. For example, consider a shape that is a composite of n individual segments, each segment having an area A_i and coordinates of its centroid as x_i and y_i. The coordinates of the centroid of this composite shape is given by

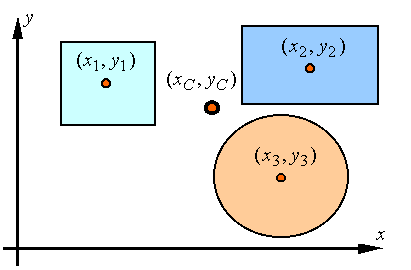
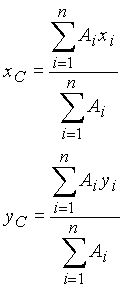
As can be seen, the location of each segment is weighted by the area of the segment and after addition divided by the total area of the shape. As such, the centroid represents the area weighted average location of the body. For a continuous shape the summations are replaced by integrations to get

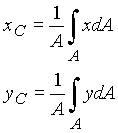
where A is the total area.

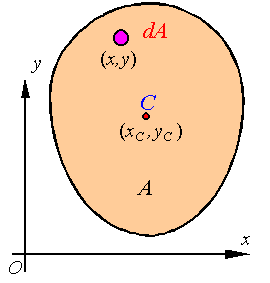
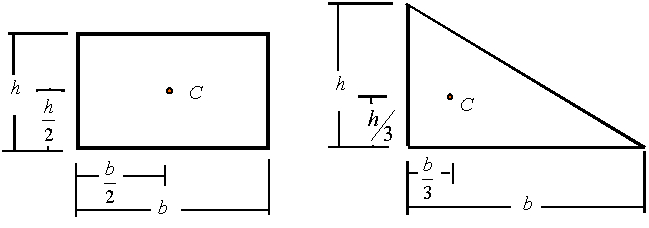
Centroid of common shapes:

The following figures show the centroid of some common objects, each indicated by a C.

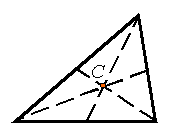
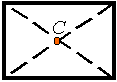
Integration of differential equations:

Differential equations are relations that are in terms of a function and its derivatives. There are some methods for solving these equations to find an explicit form of the function. Some of the simplest differential equations are of the form

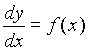
The variables in such equations can be separated to get

and then integrated to get

where C is the constant of integration. A slightly more complicated differential equation is one of the form

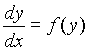
The variables in such equations can be separated to get

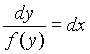
and then integrated to get

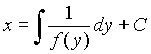
In general, if one can separate the variables, as was done in the two above examples, then one can use the methods of integration to integrate the differential equation.

For example, consider the differential equation

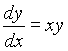
The variables in this equation can be separated to give

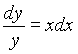
The result of integrating this expression is

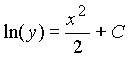
where the constant of integration can be found knowing a point (x_o, y_o) that the function must pass through. For this case

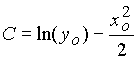
and the complete solution can be written as

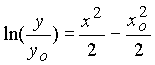
If the variables cannot be separated directly, then other methods must be used to solve the equation.