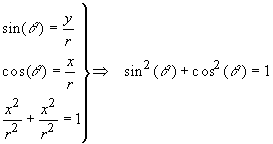TRIGONOMETRY
Areas of focus:
- Degrees versus radians
- Trigonometric functions
- Trigonometric relations between complementary angles
- Pythagorean Theorem
- The fundamental relation between sine and cosine
- The unit circle and visualizing the trigonometric functions
- Inverse of trigonometric functions
- Law of sines
- Law of cosines
- Values of trigonometric at specific angles
- Trigonometric identities
- Curves of sine, cosine, and tangent
- Approximation for small angles: sine, cosine, and tangent
One revolution is 360o, and is also 2![]() radians. Thus, due
to linear proportionality of the two scales, the conversion from x
degrees to y radians is:
radians. Thus, due
to linear proportionality of the two scales, the conversion from x
degrees to y radians is:
![]()
One radian is equal to 3.14159..., and is normally approximated by 3.14. The following table gives equivalent angles in degrees, radians, and revolutions.
|
Degrees |
Radians |
Revolutions |
|
0o |
0 |
0 |
|
30o |
|
|
|
45o |
|
|
|
60o |
|
|
|
90o |
|
|
|
180o |
|
|
|
270o |
|
|
|
360o |
|
1 |
The trigonometric functions are named sine,
cosine, tangent, cotangent, secant, and cosecant. A trigonometric
function has one argument that is an angle and will be denoted "![]() ".
In writing the trigonometric functions one uses the abbreviated forms:
".
In writing the trigonometric functions one uses the abbreviated forms: ![]() ,
, ![]() ,
, ![]() ,
,
![]() ,
, ![]() , and
, and ![]() , respectively. Also, sometimes
these are written as
, respectively. Also, sometimes
these are written as ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
and
,
and ![]() , respectively.
, respectively.
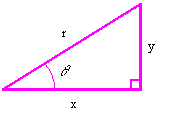
The value of each trigonometric function for an
acute angle (<90o) can be directly related to the
sides of a right triangle. Consider the angle ![]() in the following figure. The
values of the trigonometric functions for this angle are given as:
in the following figure. The
values of the trigonometric functions for this angle are given as:
![]()
![]()
![]()
![]()
![]()
![]()
Note: the
exponents of trigonometric functions follow a special rule. If the exponent "n" is positive, then
one writes ![]() in place of
in place of ![]() . For example,
. For example,
![]()
The same rule does not apply to negative exponents since the exponent "-1" is reserved for the inverse trigonometric function.
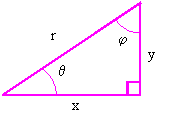
Functions of
Complementary Angles:
In this figure, ![]() and
and![]() are complementary angles,
meaning
are complementary angles,
meaning ![]() . Examination of the basic relation between the trigonometric
functions and the sides of the triangle reveal the following relations between
the complementary angles
. Examination of the basic relation between the trigonometric
functions and the sides of the triangle reveal the following relations between
the complementary angles ![]() and
and![]() .
.
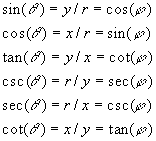
Since ![]() , we can also write:
, we can also write:
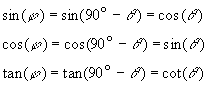
The Pythagorean theorem states that for a right triangle, as shown, there exists a relation between the length of the sides given be
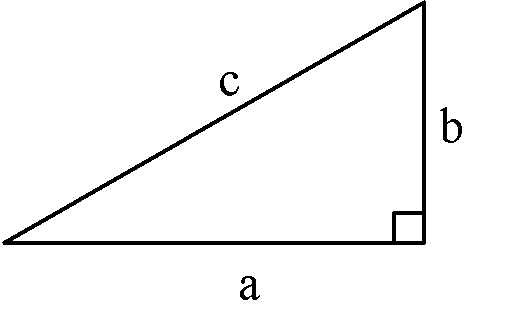
a2 + b2 = c2
There are also Pythagorean triples for (a,b,c), such as (3,4,5), (5,12,13) and (7,24,25) sided triangles, and all constant multiples of these triplets (e.g., (6,8,10)).
Fundamental
Relations Among Trigonometric Functions:
From the Pythagorean Theorem of plane geometry we know that x2 + y2 = r2. This can be used to derive a basic relation between the sine and cosine functions.