Area Moment of inertia

The area moment of inertia is the second moment of area around a given axis. For example, given the axis O-O and the shaded area shown, one calculates the second moment of the area by adding together for all the elements of area dA in the shaded area.

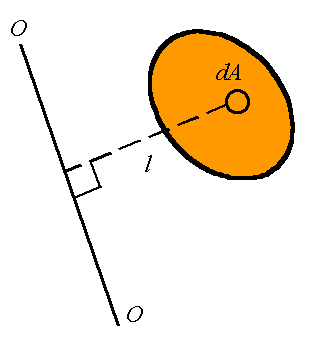
The area moment of inertia, denoted by I, can, therefore, be calculated from

If we have a rectangular coordinate system as shown, one can define the area moment of inertial around the x-axis, denoted by I_x, and the area moment of inertia about the y-axis, denoted by I_y. These are given by

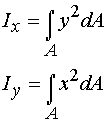
The polar area moment of inertia, denoted by J_O, is the area moment of inertia about the z-axis given by

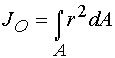
Note that since one has the relation

The radius of gyration is the distance k away from the axis that all the area can be concentrated to result in the same moment of inertia. That is,

For a given area, one can define the radius of gyration around the x-axis, denoted by k_x, the radius of gyration around the y-axis, denoted by k_y, and the radius of gyration around the z-axis, denoted by k_O. These are calculated from the relations

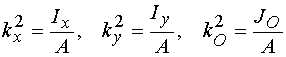
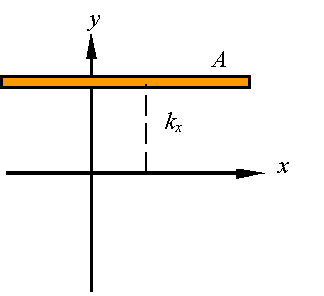
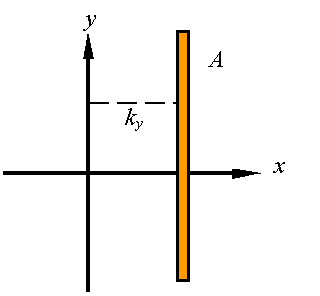
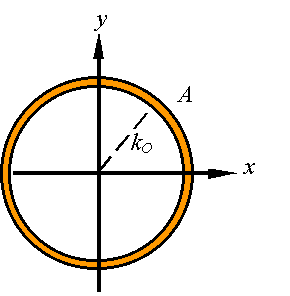
It can easily to show from that

The parallel axis theorem is a relation between the moment of inertia about an axis passing through the centroid and the moment of inertia about any parallel axis.

Note that from the picture we have

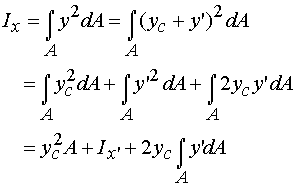
Since gives the distance of the centroid above the x'-axis, and since the this distance is zero, one must conclude that the integral in the last term is zero so that the parallel axis theorem states that

where x' must pass through the centroid of the area. In this same way, one can show that

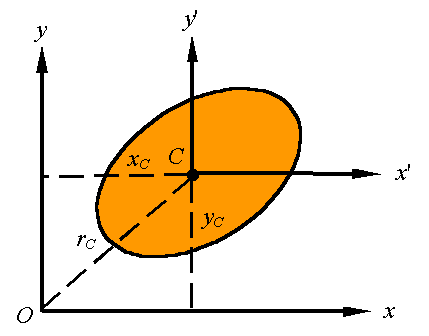
In general, one can use the parallel axis theorem for any two parallel axes as long as one passes through the centroid. As shown in the picture, this is written as

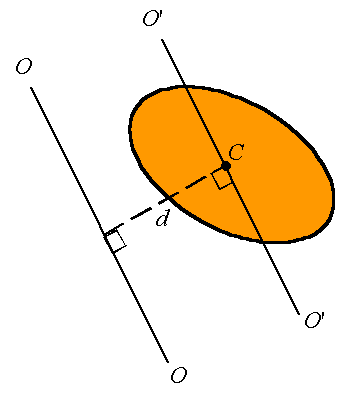
where is the moment of inertia about the axis O'-O' passing through the centroid, I is the moment of inertia about the axis O-O, and d is the perpendicular distance between the two parallel axis.

The moment of inertia of composite bodies can be calculated by adding together the moment of inertial of each of its sections. The only thing to remember is that all moments of inertia must be evaluated bout the same axis. Therefore, for example,

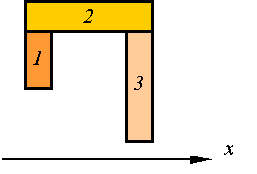
To calculate the area moment of inertia of the composite body constructed of the three segments shown, one evaluates the moment of inertial of each part about the x-axis and adds the three together.