TORSION
Basic
kinematics of torsion:
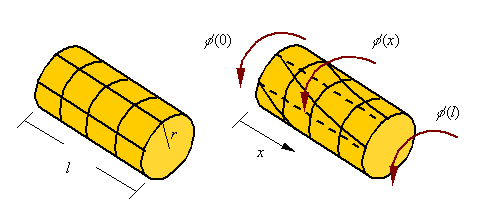
It
is assumed that when twisted each cross-section in a circular bar rotates as a
rigid body. If the amount of rotation of each cross-section is given by the
function ![]() , the total twist
, the total twist ![]() in the bar of length l
shown is given
in the bar of length l
shown is given
![]()
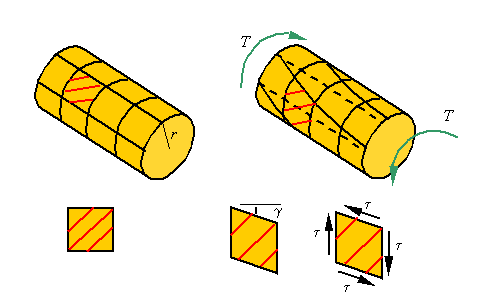
During
the torsion of a bar, axial lines drawn on the surface of a bar become helical
and circumferential lines remain circumferential. Therefore, as can be seen in
the figure, material elements are sheared in the process. This shear strain
must be accompanied by a shear stress (from Hook’s law we know![]() ).
).
The
shear strain can be related to the rotation of the cross sections. If over an
increment of length ![]() , the cross section rotates an amount
, the cross section rotates an amount ![]() , then as can be seen in the figure the shear strain is given
by
, then as can be seen in the figure the shear strain is given
by
![]()
Therefore, at the limit one can write
![]()
As a result, the shear strain starts at zero at the center and reaches a maximum at the outer radius.
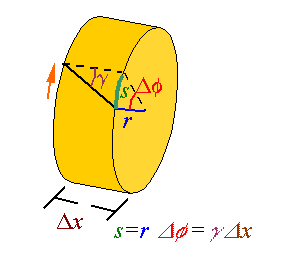
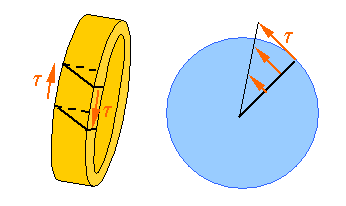
The shear stress:
The shear
stress, through Hook’s law, is related to the shear strain. Therefore, the
shear stress is given by
![]()
Again, like the
shear strain, the shear stress is zero at the core of the bar and increases
linearly to a maximum at the outer surface.
Calculating the
torque from the shear stress:
The torque transmitted through the cross-section of a bar is the resultant of the moments created by the shear stresses on the cross-section.
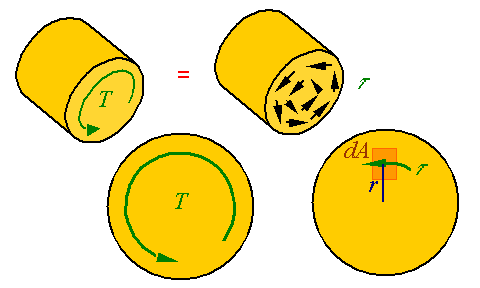
The differential shear load dV
that results from the shear stress applied over the area dA of the cross
section is ![]() . The moment created by such a shear load is dM = rdV.
The equivalent torque T on the cross section is the resultant of all the
moments crated by the shear stresses so that
. The moment created by such a shear load is dM = rdV.
The equivalent torque T on the cross section is the resultant of all the
moments crated by the shear stresses so that
![]()
Using the expression for shear stress in
terms of twist in the bar we get
![]()
where Ip is the polar
area moment of inertia of the cross section. Using this relation, the
expression for shear stress in terms of the torque follows from
![]()
Total twist in
a bar:
The total twist in a bar is given by
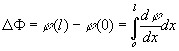
The twist per unit length in terms of
the torque is given by
![]()
Therefore, the total twist can be
calculated from
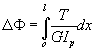
In the case of a uniform bar for which the ![]() is constant one can move the argument out and integrate to
get
is constant one can move the argument out and integrate to
get
![]()
ã Mehrdad Negahban and the University of Nebraska, 1996-2000.
All rights reserved
Copy and distribute freely for personal use only
Department of Engineering Mechanics, University of Nebraska, Lincoln, NE 68588-0526